The motion of ions is not important for describing the whistler wave radiation in the chorus excitation region with frequency ω in the interval ${\mathit{\omega }}_{\mathrm{LHF}}<\mathit{\omega }<{\mathit{\omega }}_B<{\mathit{\omega }}_{\mathrm{p}}$, where ω_LHF is the lower-hybrid frequency, ${\mathit{\omega }}_B=eB/mc$ and ${\mathit{\omega }}_{\mathrm{p}}=(\mathrm{4}\mathit{\pi }{n}_{\mathrm{p}}{e}^{\mathrm{2}}/m{)}^{\mathrm{1}/\mathrm{2}}$ are the absolute value of the electron cyclotron and plasma frequencies, e is the absolute value of electron charge, m is the electron mass, n_p is the plasma density, and c is the speed of light in free space. A theoretical analysis is simplified if the conditions for the applicability of the so-called quasi-longitudinal approximation of electromagnetic wave propagation in a cold, relatively dense plasma are fulfilled (Helliwell, 1965):

where k_z and k_x are the wave vector components along and across the magnetic field, respectively.

For typical magnetospheric conditions (Haque et al., 2011) in the region of chorus excitation, the length of the whistler wave λ≃15 km is less than the scale of the background plasma density distribution and the transverse size of the duct, d = 100–300 km. Therefore the inequality $\mathit{\lambda }/d\ll \mathit{\pi }$ is fulfilled, and the well-known WKB approximation (Budden, 1985) is valid. When the plasma density is inhomogeneous in the direction perpendicular to the duct axis, refraction is an important factor for the wave propagation. It is known that under conditions for refractive reflection, waves with frequencies higher than half of the electron cyclotron frequency are directed by the depleted duct (Helliwell, 1965). Waves with frequencies lower than half of the electron cyclotron frequency are directed by the enhanced duct (Helliwell, 1965; Karpman and Kaufman, 1984).

We now consider in more detail the characteristic equation of a refractive planar duct. For this purpose, we analyse the dependence of the transverse component of the whistler wave vector on the frequency and the longitudinal component of the wave vector with different background plasma densities (Woodroffe et al., 2013). This dependence, in accordance with Fig. 1, has two branches, which, according to Eq. (1), can be written as follows:

where $u_{\mathrm{G}}=(c{\mathit{\omega }}_B/\mathrm{2}{\mathit{\omega }}_{\mathrm{p}})$ is the Gendrin velocity (Helliwell, 1995),

H(ς) is the Heaviside step function. Let us note that there is a range of values k_z, ω, in which k_x has not one but two values (see Fig. 1).

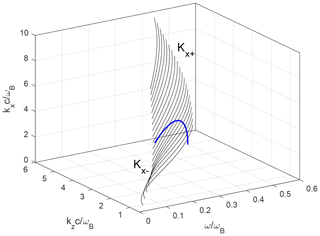
Figure 1Dependence of the transverse component of the wave vector on the frequency and the longitudinal component of the wave vector for $({\mathit{\omega }}_{\mathrm{p}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{25}$ are shown by contours with constant k_x in the surface $k_x=k_x(\mathit{\omega },k_z)$. Two branches K_x− and K_x+ (see Eq. 2) are separated by a blue line.

Download

The property of the eigenmode wave solutions is determined by the characteristic equation, which expresses the dependence of the frequency on the longitudinal component of the wave vector. For determining the characteristic equation in the WKB approximation, it is necessary to calculate a complete transverse change in the phase during the ray propagation period. A change in the phase during one period should include phase displacements on the caustics and be a multiple of 2π. Therefore, the characteristic equation takes the well-known form (Laird, 1992)

where p is a positive integer, and in a symmetric duct $\mp x_{max}$ values are the reflection levels, where k_x=0.

Within the framework of the WKB approximation, the dependence k_x(x) should be continuous, and in the regions of refraction reflection, k_x=0 if the density n_p(x) and the Gendrin velocity u_G(x) change continuously. The refraction-enhanced duct can occur for frequencies $\mathit{\omega }<{\mathit{\omega }}_B/\mathrm{2}$, and only branch K_x− (Eq. 2) satisfies the continuity condition of the dependence k_x(x). The refraction-depleted duct can occur for frequencies $\mathit{\omega }>{\mathit{\omega }}_B/\mathrm{2}$, and only the branch K_x+ (Eq. 2) satisfies the continuity condition of the dependence k_x(x).

Let us assume that there is a duct with enhanced or depleted cold plasma density and which is uniform along the magnetic field. While performing calculations, we will consider, according to the known experimental data (see, e.g. Hague et al., 2011; Taubenschuss et al., 2014; Agapitov et al., 2017), that the magnetic field is uniform and that the electron cyclotron frequency is ${\mathit{\omega }}_B=\mathrm{6}\times {\mathrm{10}}^{\mathrm{4}}$ s⁻¹. The plasma density outside the duct corresponds to the condition $({\mathit{\omega }}_{\mathrm{p},\mathrm{out}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{25}$. Inside the enhanced duct, we have $({\mathit{\omega }}_{\mathrm{p},\mathrm{int}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{29}$, while inside the depleted duct, $({\mathit{\omega }}_{\mathrm{p},\mathrm{int}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{21}$. For determining the solutions of the characteristic equation, we used a model transverse density distribution for the enhanced and depleted ducts in the form

where d = (1–3) 10⁷ cm is the transverse size of the duct.

For the accepted density distributed across the duct (Eq. 4), it is possible to numerically calculate, based on Eq. (2), the phase change given by Eq. (3) and obtain dispersion formulas for the enhanced and depleted ducts. Some results of calculations are given in Fig. 2. In this figure, the red lines show solutions of the characteristic equations for the first and seventh modes in the enhanced (panel a) and depleted (panel b) ducts, the dashed–doted and the dotted curves correspond to the solution of dispersion Eq. (1) for k_x(x)=0 at the maximum and minimum density, respectively, the solid line corresponds to the Gendrin velocity at the maximum density, and the dashed straight line corresponds to the Gendrin velocity at the minimum density.

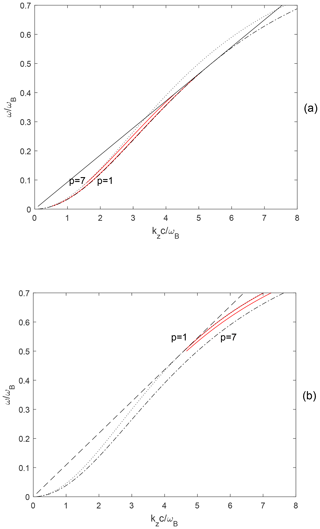
Figure 2Relationship between the frequency and the longitudinal component of the wave vector for the first (p=1) and seventh (p=7) ducted modes at $({\mathit{\omega }}_{\mathrm{p},\mathrm{out}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{25}$ are shown in red: (a) in the enhanced duct with $({\mathit{\omega }}_{\mathrm{p},\mathrm{int}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{29}$ and (b) in the depleted duct with $({\mathit{\omega }}_{\mathrm{p},\mathrm{int}}/{\mathit{\omega }}_B{)}^{\mathrm{2}}=\mathrm{21}$ .

Download

For relatively gently sloping ray trajectories, it is not difficult to estimate the average of the normal angle. Then Eq. (3) can be written in the form $(\mathrm{2}/d)\underset{\mathrm{0}}{\overset{x_{max}}{\int }}\tan \left(\mathit{\theta }\right)\mathrm{d}x=(\mathrm{2}\mathit{\pi }/k_{z}d)(p-\frac{\mathrm{1}}{\mathrm{2}})$. As it will be shown in the next section $\mathit{\theta }<\mathit{\pi }/\mathrm{4}$ for actual modes, with an accuracy of up to 20 % we have tan (θ)≃θ , and therefore

Note that the distance between the modes in Fig. 2 decreases with increasing transverse size of the duct.

Now we briefly recall the process of chorus frequency–time spectrogram formation in the implementation of the BPA mechanism (Bespalov and Savina, 2018). At the input of the wave–particle interaction region (z=0), there is weak noisy emission with an electric field containing a random sequence of weak shot noise electromagnetic pulses. Noise that does not satisfy the conditions for interaction with particles is dumped in a nearly stable plasma. An appropriate shot pulse is a trigger for the chorus discrete element. In accordance with this, we assume that a short noise pulse flies in the duct on the z axis along the magnetic field B. Our interest is to classify the duct solutions which are close in structure to the expression

in which the longitudinal electric field E_z corresponds to the pth wave mode in the duct. The wave mode (Eq. 6) is localised well on the transverse coordinate x because of the fast decrease in the function G_p(x) with large |x|, but in the general case, it spreads along the longitudinal coordinate z because of the dispersion.

There is an important exception from this general regularity. A noisy electromagnetic pulse will not rapidly spread if it has frequencies for which

where $v_{phz}=\mathit{\omega }/k_z$ and $v_{gz}=\partial \mathit{\omega }/\partial k_z$ are the phase and group velocities of the wave mode in the duct. In this case, the population of epithermal electrons, which fly in together with the pulse into the region of wave–particle interaction, starts to play an important role. These electrons for the pulse are a single-velocity beam with a distribution function f proportional to

where δ(ς) is a delta function, n_b is the density of the beam coordinated with the pulse, and V_z is the beam velocity.

In a homogeneous plasma with beam (Eq. 8), the electromagnetic pulse increases with the growth rate (Bespalov and Savina, 2018):

First of all, we verify the possibility of the fulfilment of conditions (7) for the duct modes. For the plasma parameters introduced in the foregoing section, this condition can be fulfilled (see Fig. 2a) in the enhanced duct if the frequency spectrum is concentrated near $\mathit{\omega }/{\mathit{\omega }}_B\simeq \mathrm{0.42}$ and the longitudinal component of the wave vector is close to $k_{z}c/{\mathit{\omega }}_B\simeq \mathrm{4.7}$. Conditions (7) also can be fulfilled (see Fig. 2b) in the depleted duct if the frequency spectrum is concentrated near $\mathit{\omega }/{\mathit{\omega }}_B\simeq \mathrm{0.51}$ and the longitudinal component of the wave vector is close to $k_{z}c/{\mathit{\omega }}_B\simeq \mathrm{5.0}$. The mentioned frequencies are typical for lower- and upper-band chorus (Lauben et al., 2002).

We can use a formula for growth rate (Eq. 9), since the wave modes in the duct conform to the WKB approximation, and therefore their polarisation corresponds to the whistler waves in which during the inclined propagation there is a longitudinal component of the wave electric field which is taken into account in the growth rate calculation (Bespalov and Savina, 2018). We will refine now the value of the average angle $\stackrel{\mathrm{‾}}{\mathit{\theta }}$, which should be substituted into Eq. (9). We will do this using Eq. (5), according to which

where we expected that $k_{z}c/{\mathit{\omega }}_B\simeq \mathrm{5}$ and d=300 km. The angle θ is less than 20^∘ for the first 10 modes.

Actually, the number of operating modes (p) in a relatively small duct is limited by several factors. In particular, the quality of the mode should be sufficiently high, the mode should be electromagnetic, and the key conditions (7) should be fulfilled. Formally, the growth rate (Eq. 9) increases with an increasing mode number p. However, it should be taken into account that there is another limitation on the angle of excitation, which is caused by the Landau damping on the Cherenkov resonance of electromagnetic waves in a plasma. We note that for the angle θ=20^∘, the growth rate (Eq. 9) decreases by 20 % only in comparison with the maximum value close to θ=39^∘, and it is sufficient for explaining the experimental data.

The paper is theoretical and no new experimental data are used. All figures data are obtained from numerical calculation in MATLAB codes. Corresponding parameters are listed in the text.

PAB proposed and analysed the BPA mechanism in the density ducts and wrote the paper. ONS analysed the BPA mechanism in the density ducts and wrote the paper.

The authors declare that they have no conflict of interest.

This research has been supported by the RSF (grant no. 16-12-10528).

This paper was edited by Wen Li and reviewed by two anonymous referees.